Grade 3: EDC Counting Tape – Beginning of the School Year

Instructor Notes:
For materials preparations, click again on Samples and select Getting Started 3.
Read Every Day Counts Teacher’s Guide, Kanter and Gillespie, Great Source, 2005 or 2012 edition, p. 23-25.
Before Day 13: On some of the even-numbered Days of School, after attaching a yellow Post-It displaying the number, you might have this number of students stand and pair up to show how even numbers break up into groups of two with no leftovers or remainders. On some of the odd days like Day 13, doing the same activity, students can see how an odd number, when divided into pairs, results in one extra without a partner.
To help children learn to recognize even and odd numbers, you might want to record a vertical list of even numbers and circle the digits in the ones place to examine the 2,4,6,8, 0 pattern. Doing the same with the odd numbers may help students see the 1,3,5,7,9 pattern in the ones place. This can lead to exploring why the digit in the one’s place determines if a number is even or odd?
On the Hundred Chart, circle the new Day of School and then choose the number of an earlier day to circle, also. Invite the class to compute the difference and ask volunteers to share their various strategies. For example, for 13 – 8, one strategy might be, “It takes 2 to get to 10 and 3 more to 13. That’s 5.” This “take it out of the ten” strategy can help students who may still be working to master the subtraction facts.
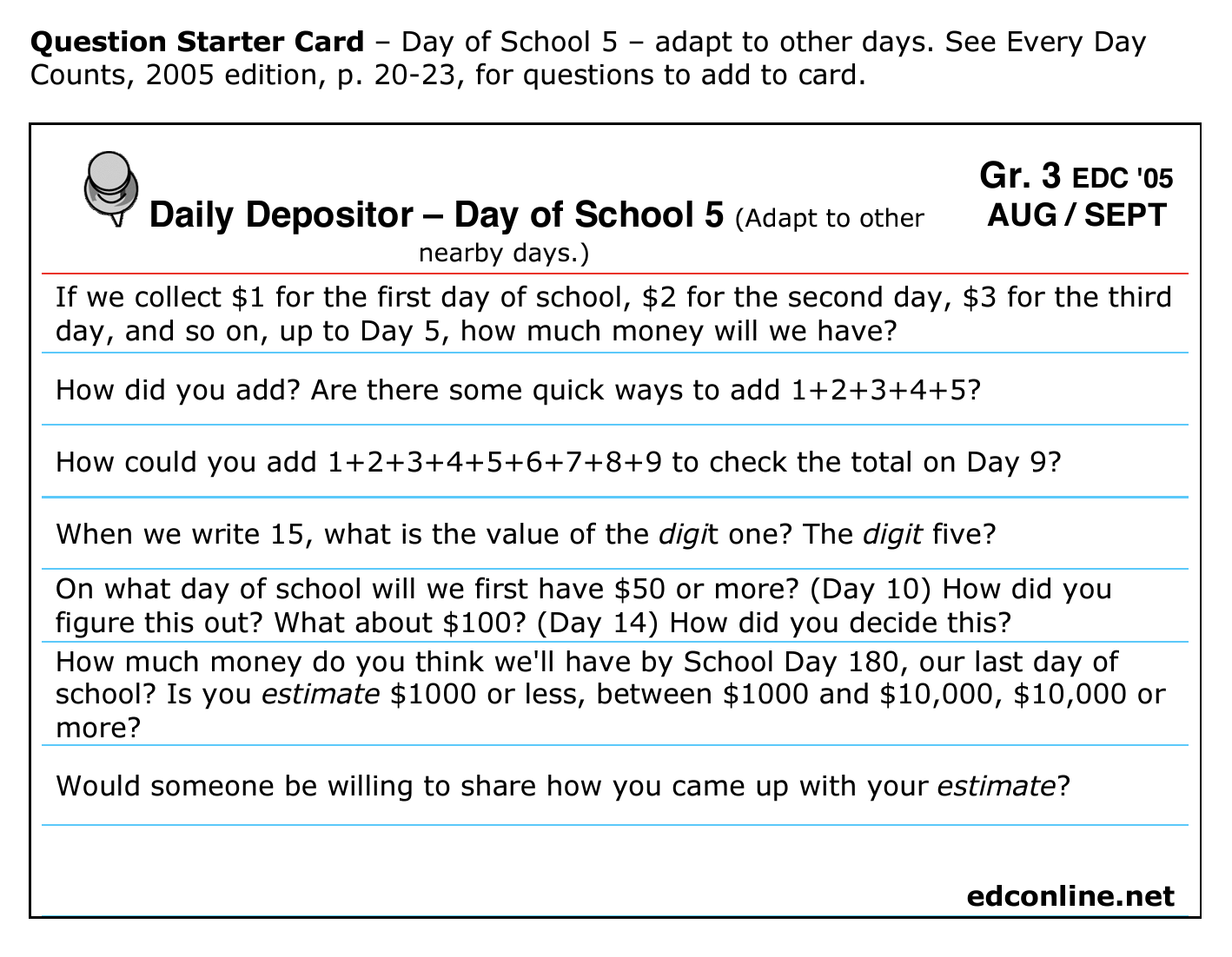
Grade 3: EDC Daily Depositor – Beginning of School Year

Instructor Notes:
For materials preparations, click again on Samples and select Getting Started 3.
Read Every Day Counts Teacher’s Guide, Kanter and Gillespie, Great Source, 2005 or 2012 edition, p. 20-23.
Day of School 5: Since the number of the Day of School is used to determine the amount added to the Depositor each day in Grade 3, it is helpful to introduce it right away. On Day 5, you might first review how the money is being collected.
Invite one child up to hold one dollar for the first day of school, a second child to hold two dollars for the second day, a third child to hold three dollars, a fourth to hold four dollars, and a fifth to hold five dollars for the fifth day of school. Have students predict the total for Day 5. Then encourage sharing of the various ways they added to get $15. Let the five students holding the money decide how they can combine their amounts to come up with ten and trade for a $10 bill. Deposit the ten-dollar bill and the five extra ones into the Depositor pockets and record digits above each pocket to show the total.
You might engage the class in making some predictions and to share their reasoning. What day do you think we’ll reach $50? $100? $1000?
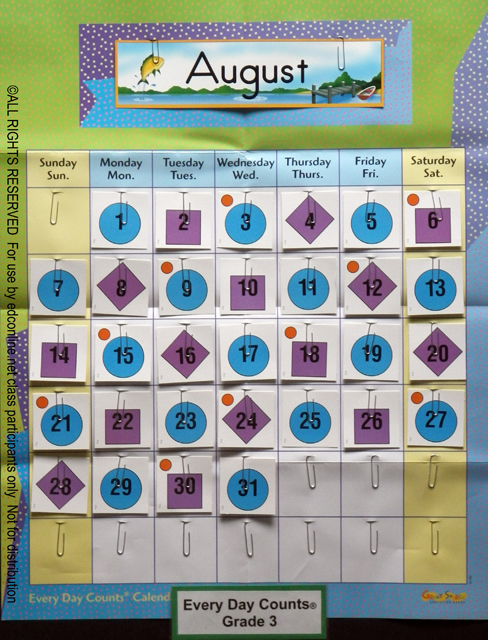
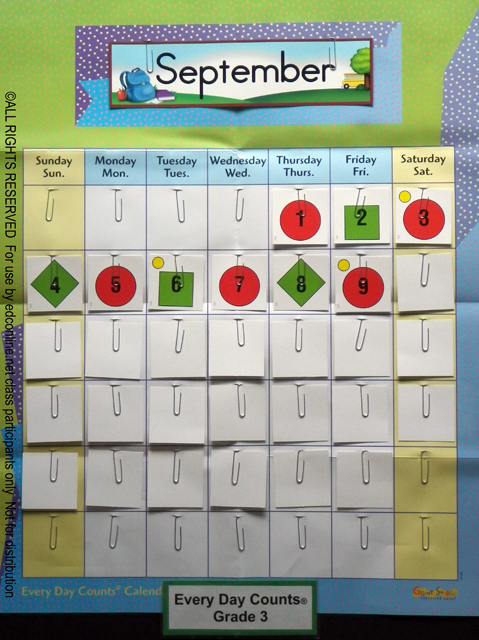
Grade 3: EDC Calendar – Beginning of September or 1st Month of School
Instructor Notes:
For materials preparations, click again on Samples and select Getting Started 3.
Read Every Day Counts Teacher’s Guide, Kanter and Gillespie, Great Source, 2005 or 2012 edition, p. 18-20.
If you are on a year round schedule or begin school in August, you may want to use the August calendar pieces for your first month of school. Both the August and September pieces present an ab odd/even pattern using circles and squares with a dot in the corner of every third piece.
To introduce the calendar, have the class read the days of the week in order across the top and place the piece for the first day of the month in the correct space. Let children name its day of the week and its date. Catch up to the present date.
Invite the class to name the shapes, so everyone notices the circle square pattern. Children can discuss the question, “Is a square still a square when it is turned on end?” On several days during the month, you may want to have a number of students equal to the date stand and pair up to demonstrate that the number is odd or even. Students can see that when pairs come out evenly, the amount is an even number and when there is one student left out, it’s odd.
Later in the month we can ask students to predict and describe a future piece. Encourage everyone to explain his or her reasoning.
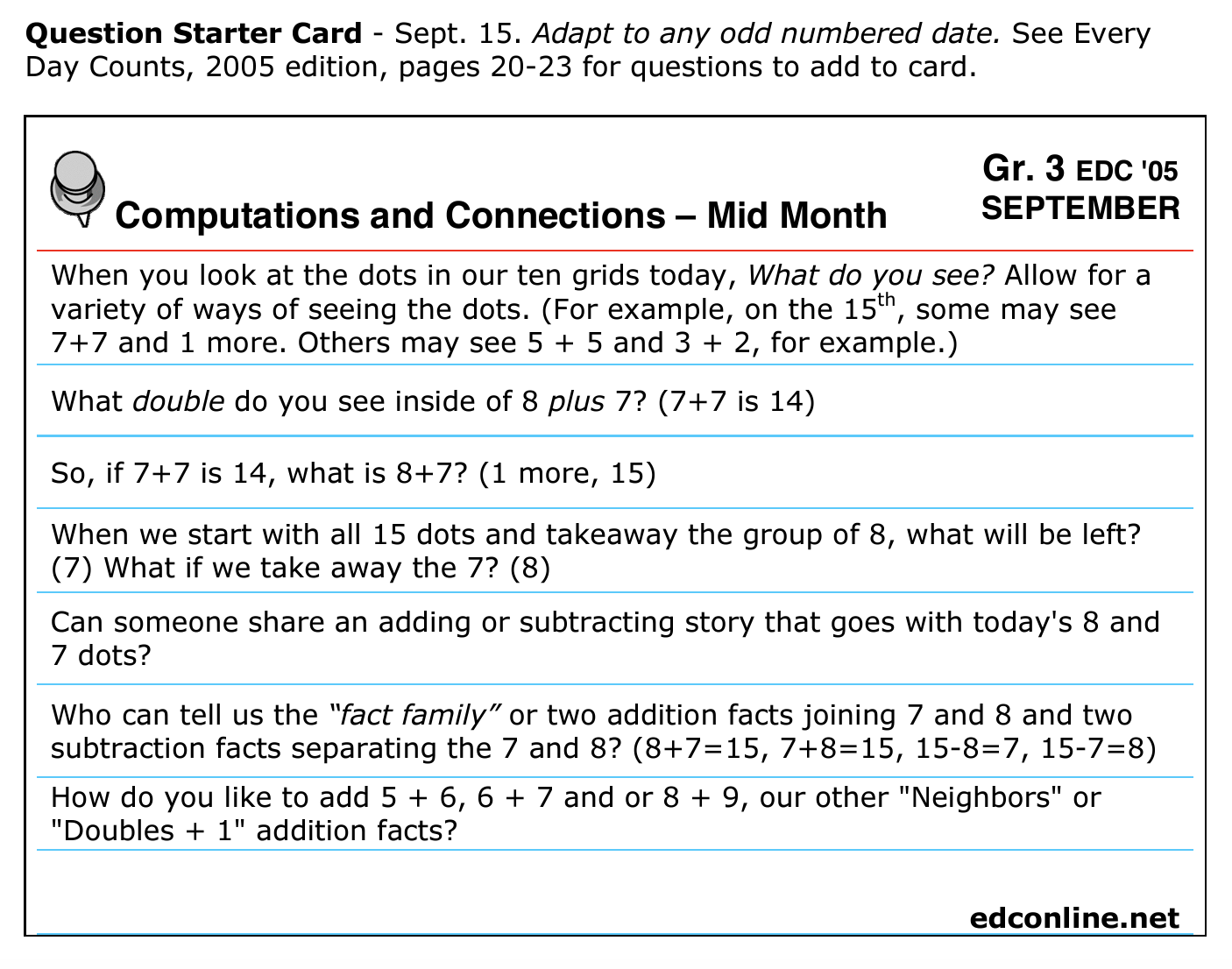
Grade 3: EDC Computations & Connections – Middle of the Month

Instructor Notes:
For materials preparations, click again on Samples and select Getting Started 3.
Read Every Day Counts Teacher’s Guide, Kanter and Gillespie, Great Source, 2005 or 2012 edition, p. 20-23.
On the odd numbered days in September when a “neighbor ” or ” double plus one” fact appears in the double ten grid, children may need help seeing the more familiar double within. For example, on September 15th, when looking at 8+7 or 7 + 8, we can ask, ” What was the double before we added one more? “When children recognize a fact as a “neighbor” or “double plus one” they often find they can use the easier “double” fact to help them with these “harder facts”.
Other Strategies : When looking at the double ten grids, some students may suggest other ways of working with 8 and 7 to get 15. Some may suggest taking two from the seven to give to the 8, making the 8 into a full ten with five left to make 15. Others may suggest “pulling out five” from 8 and from 7 to make ten, and adding on the leftover 3 and 2 to make 15. It is exciting when children begin to develop this “friendliness with numbers”, to quote Bob Wirtz. He promoted the use of ten grids and other visual models to foster number sense and fact mastery over 30 years ago.
Grade 3: EDC Probability Graph – September

Instructor Notes:
For materials preparations, click again on Samples and select Getting Started 3.
Read Every Day Counts Teacher’s Guide, Kanter and Gillespie, Great Source, 2005 or 2012 edition, p. 28-30.
By early in September we can look at the Calendar’s ab color pattern and see that half of the pieces are red and half are green on even numbered days. Placing two red circles and the two green squares that match the color pattern (without numbers) into a lunch sack and drawing and replacing them a total of 60 times over the month gives children the chance to predict the outcome based on the chances of drawing each color. Graphing the results of 15 draws (or an even-numbered 20 draws) at a time allows students to compare the cumulative data against the fact that reds and greens are equally likely to be drawn. Are there some surprises? As the sample increases will the results come closer to the probability of 1/2 red and 1/2 green?